BME Géza Pattantyús-Ábrahám Doctoral School

Department: Department of Polymer Engineering

Supervisor: Dr. Marianna Halász

 

Analysis of 3D deformation of woven composite reinforcements

Introducing the research area

Earlier, fabrics were only used to produce traditional garment and home textiles, but nowadays it took place as reinforcements of the light and, at the same time, strong composites. As reinforcements, mainly woven structures are used because their fiber orientation is known, and hence the deformation of the finished product can be designed easily unlike other fabric structures. The mechanical and structural properties of fabric reinforced composite are influenced mainly by the properties of fabric. Due to the special properties of fabrics, the modeling of 3D deformation is still problematic in all areas of application.

Brief introduction of the research place

In my work, I continue with the textile profile of Department of Polymer Engineering. The Department was the among the first universities in Hungary to introduce MSZ EN ISO 9001:2009 quality control system. Later, instruments of the laboratory was accredited by the Hungarian Accreditation Board (NAT). eXPRESS Polymer Letters, the journal of the Department is published monthly in English language.

History and context of the research

 

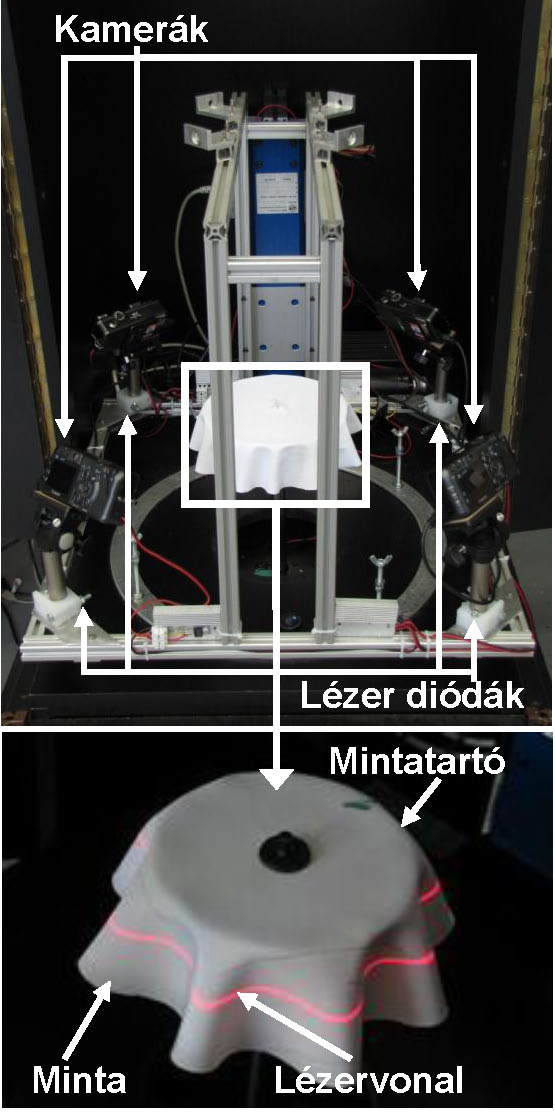
In the last decades, application of woven fabrics as composite reinforcements directed focus of studies to fabrics deformability. The first composite products appeared in the fifties in aircraft parts. Having discovered their favorable properties, i.e. the high strength is combined very low weight, composite materials have become wide spread. Reinforcements were first made from glass, carbon and aramid but then basalt and natural fibers appeared as well. Composite products frequently have complex geometry, which underlies the importance of design of strength and deformability. The goal of these studies is to know the deformation process and also to assess whether it will properly fit on the geometry – an important factor influencing the mechanical properties of finished product [1-5]. Drapability characterize globally the 3D deformation of fabrics [6-7]. At first, those properties were determined manually but later the Department of Polymer Engineering jointly with the Department of Mechatronics, Optics and Engineering Informatics developed the modern, image processing-based Sylvie 3D Drape Tester which calculates results automatically [8].

Sylvie 3D Drape Tester

The research goal, open questions

The goal of my study was to examine the mechanical and structural properties of woven fabrics and to analyze the relation between them. Knowing the parameters influencing deformability allows simulating processing, finite element modeling and virtual display. My related goals in more details are as follows:

 

1. Examine bending and shear properties of woven fabrics, because these are the properties that influence mostly 3D deformation. Furthermore, developing test methods to study those parameters.  

2. Study friction between yarns in the woven fabrics and analyze its impact on fabric drapability.

3. Analysis of yarn’s twist direction and its effect on drapability.

4. Development of a drape tester to help analyzing the reason of large variability in the extent of drapability .

 

For my studies, I choose 2 woven fabric made of glass, 1 carbon and 1 aramid woven fabric, whereas from the area of traditional fabrics 3 cotton and 1 polyester fabric were chosen.

 

   

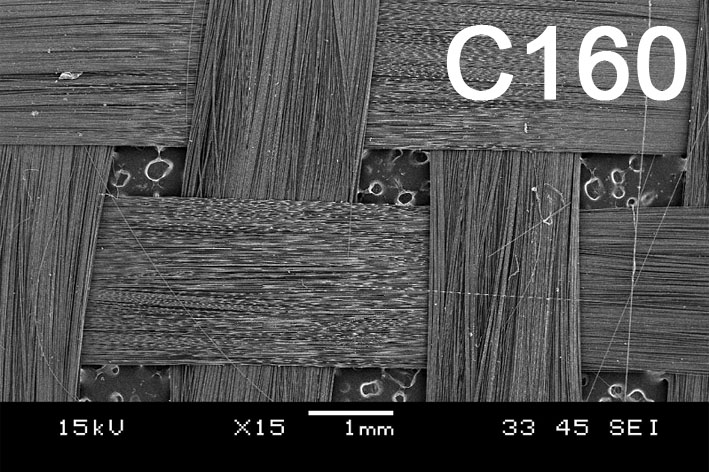
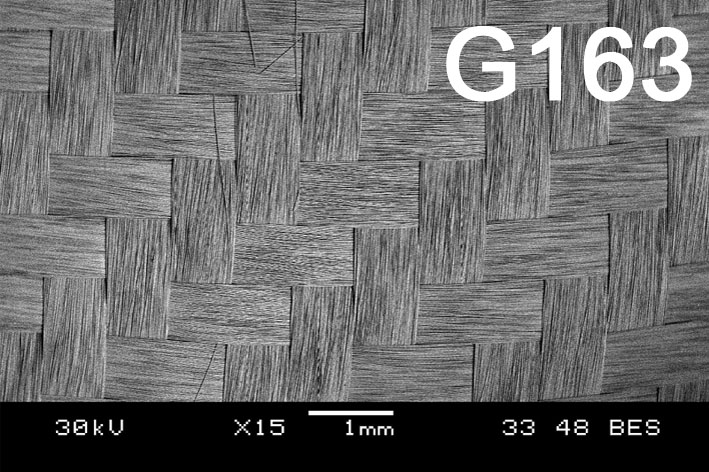
SEM pictures of reinforcements

 

 

Note that the 3 cotton fabrics were specially made for analyzing yarn twist direction effect on drapability, so these fabrics differ only in their yarns twist direction.

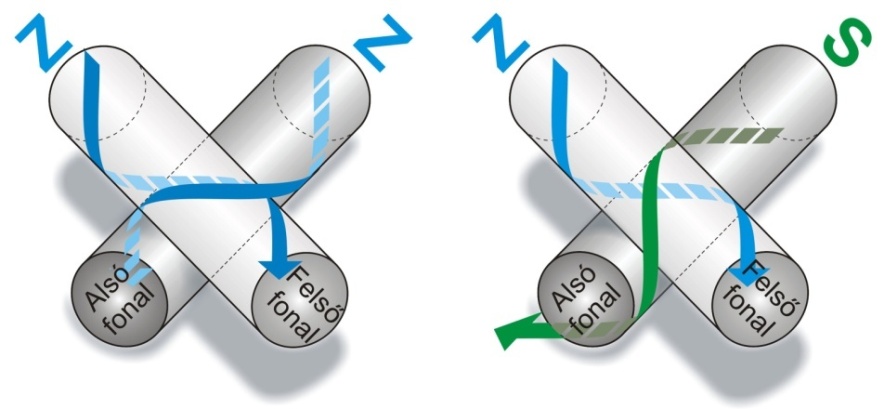
Twist direction of yarns and their connection

  

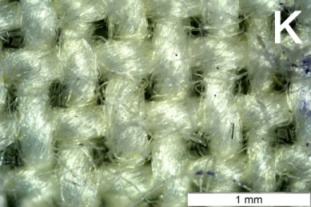
Pictures of cotton fabrics by microscope,

Twist direction are the same in warp and weft yarns are the same in P (S-S ), alternating in F (S-S/Z), different in K

 

Methodology

Drape measurement

 

Drapability can be characterized generally by drape coefficient, which is the ratio between the projected area of the plane and that of the draped fabric. Drape tests were made by Sylvie 3D Drape Tester.

The computer-controlled equipment first scans the 3D surface of the sample, and then the software reconstructs the form and calculates drape parameters.

 

New method of drape measurement

 

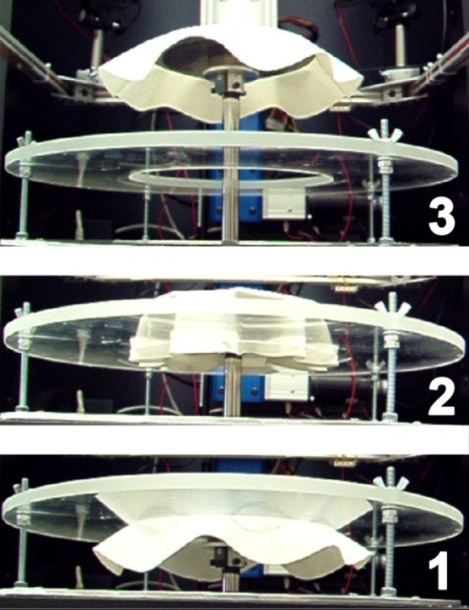
Fabrics drapability can easily be changed even by a small external force, and will switch to a new stable state. To assure that these influences are definite and reproducible, I completed Sylvie 3D Drape Tester with rings of various diameters to influence formation of drapes. In operation, the sample holder when lifted will push the sample through the ring thus influencing drapability and will result in various shapes. As a result, application of the ring will decrease of impact of random external effects on drape formation.

Sylvie 3D Drape Tester completed with a ring

Bias extension test

 

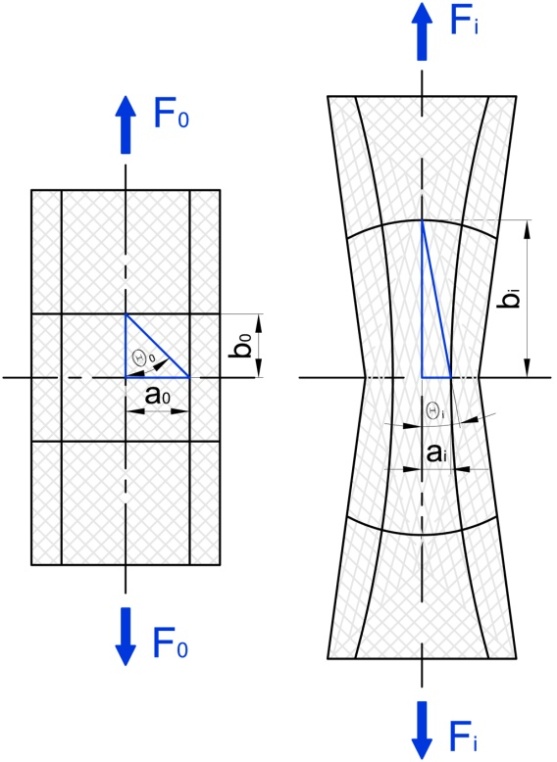
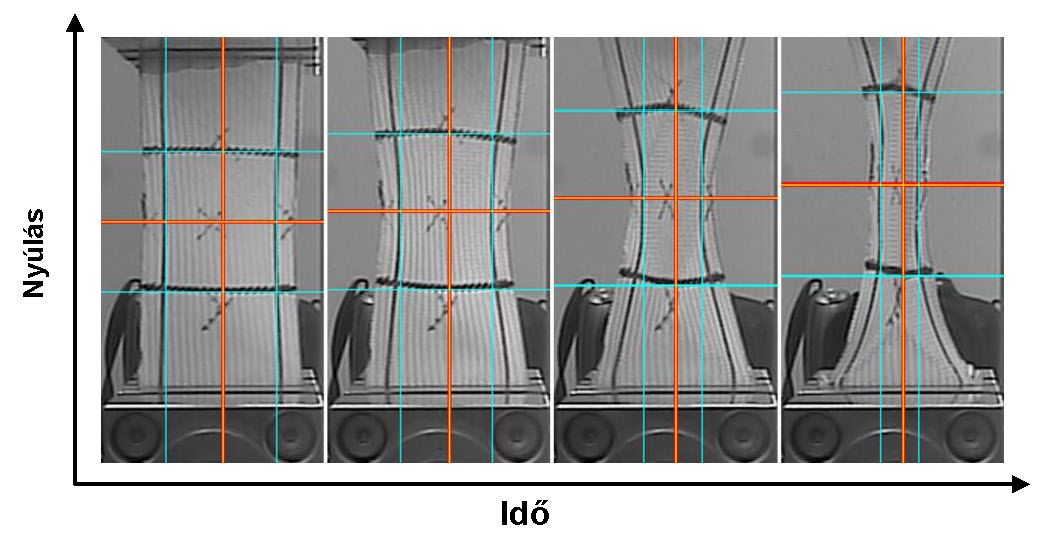
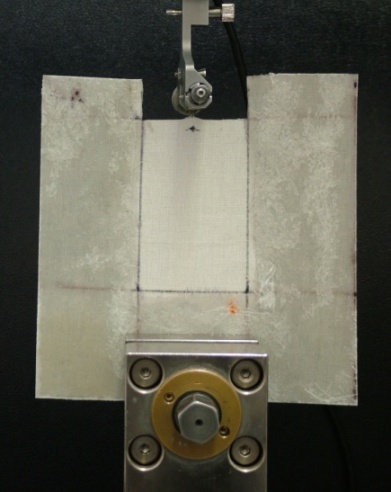
During bias extension, shear force and shear deformation are the determined from the tensile tests of the sample cut from the fabric at a ±45° angle compared to the weft direction. Shear deformation is often characterized by the change in the angle of the fabric yarns, and is called shear angle, usually denoted by γ. A new method was developed for the evaluation of bias extension tests that enables determination of shear angle in a more simple and accurate way than described above. An important element of the new method is that additionally to the longitudinal elongation of the examined sample the decrease of cross directional width is also used for the calculation of the shear angle. The dimensional change of the sample is determined by an videoextensometer, and for this purpose longitudinal and crosswise lines were drawn on the sample before the examination. These lines from a rectangle in the pure shear zone, and a square in case of an ideal sample. The sample is ideal if the angle between the warp and weft yarns in the fabric is 90° before the test. The lines have to be drawn so that the centers of the sides of the rectangle or square form a rhombus or in case of an ideal sample a square the sides of which are parallel with the yarns of the fabric. During the tests, the actual distance of these lines at the locations defined in the longitudinal and crosswise center lines of the sample are recorded.

.

 

Marked fabric samples to determine shear angle in loaded and unloaded state. 2ai width decreasing 2bi length increasing 2Θ angle between warp and weft yarns

Photo series about deformation of sample under bias extension

 

Yarn pull out test

 

Yarn-yarn friction can be determined by using Euler equation [9], for that result yarn pull out test and the central angle of yarn in fabric are needed. Adequate clamping of the sample had to be solved before starting the measurement and any undesired slipping of the fabric had also to be prevented. Both problems were solved by forming composite parts on the sample. The hatched parts that were prepared by manual lamination using glass fiber mats and polyester resins in 50 mm thickness on both sides, and on the top and bottom. The laminated bottom of the sample is clamped with a general, 60 mm wide clamping jaw, and the yarn to be pulled out is clamped with a yarn clamper on the top, while the laminated bands provide the lateral clamping automatically.

Yarn pull out measurement

 

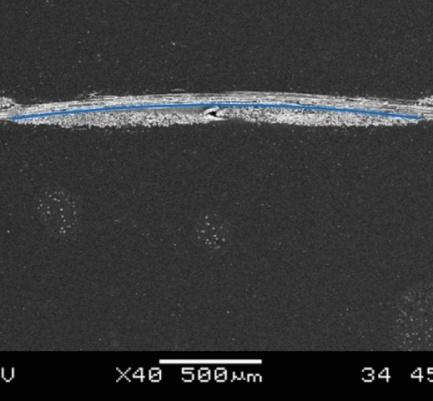
Determination of yarn’s central angle

Where warp and weft yarns cross each other they get curved in the woven fabric. The central angle belonging to the curve was determined by taking images of the cross section of fabrics by SEM.

 

Results

Effects of rings

 

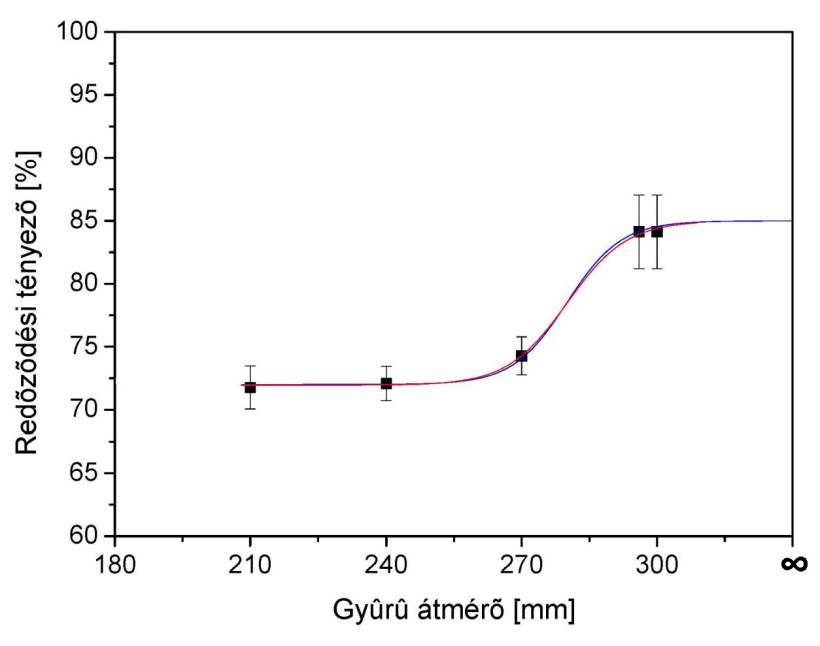
I concluded that the drape method I developed offers a better approximation of the real conditions of use than traditional methods, i.e. those without rings. Furthermore, it also increases the drapability of the sample, i.e. decreases drape coefficient and its standard deviation. The change in drape coefficient by the effect of the ring follows the logistic curve. Drape unevenness decreases, too.

The fitted curve for cotton fabrics

 

D210	D240	D270	D300

Drapability using different ring diameters

 

Effect of yarn test direction

 

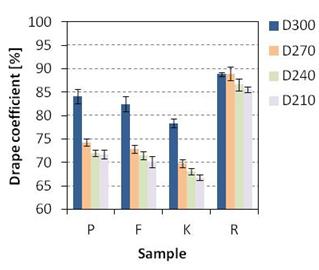
Drape coefficient and shear rigidity are bigger, thickness are smaller in case of same yarn twist direction compared to different yarn twist direction.

Drape coefficient by fabric type using different ring diameters

 

Bias extension test

The new method I developed can evaluate bias extension test results and determine shear angle by the simple equation. The method is more precise then other methods and it is simpler, because it can be used only with tensile tester and videoextesometer without any other instrument.

 

 

 

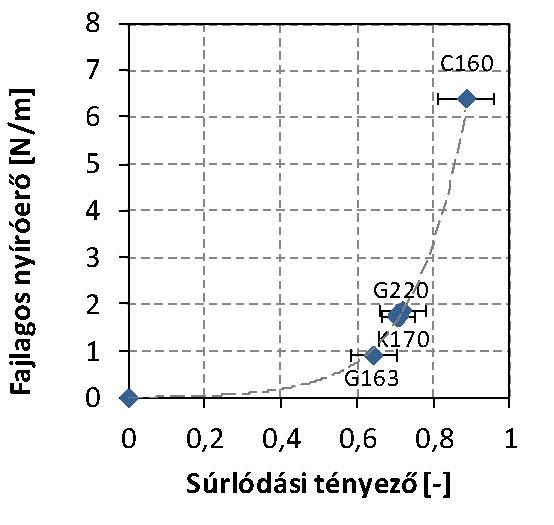
Effect of friction

In my study I found that the relationship between friction and specific shear force can be approximated with exponential equation in the measured range in the case of reinforcements.	Specific shear force - friction at reinforcements
Drape coefficient difference – friction at reinforcements	The relationship between yarn-yarn friction and the difference of drape coefficient of traditional and drape coefficient measured by ring with 210 mm inner diameter follow exponential equation in the measured range in the case of fabric reinforcements.

Expected impact and further research

There are many possibilities in the analysis of 3D deformation properties of woven fabrics.

For example, parameters influencing 3D deformation of woven fabrics can be further analyzed by biaxial extension tests, therefore I plan to develop an equipment for that.

A further research field can be development of equipment to measure bending properties of reinforcements.

Using samples with larger diameter for drape tester in case of fabric reinforcements is also beneficial because of the higher rigidity and will provide better drape characteristics. This will bring along the development of the equipment, too.

 

Publications, references, links

Publications

 

1. Al-Gaadi B., Göktepe F., Halász M.: New method in drape measurement and application of results in modeling of fabric behavior. Textile Research Journal, 82, 502-512 (2012). (IF: 1,102)
2. Al-Gaadi B., Halász M.: Analysis of shear behaviors of woven fabrics with image processing. ‘International Joint Conference on Environmental and Light Industry Technologies, Hungary, Budapest’ 2010 11. 18-19. 6 pp (Presentation and CD).
3. Göktepe F., Halász, M., Tamás P., Göktepe Ö., Gersak J., Al-Gaadi B., Özdemir D.: Twist direction and yarn type effect on draping properties. ‘International Symposium on Novelties in Textiles, Slovenia, Ljubljana’, 2010. 05. 27-29. 171-177 (Presentation and CD).
4. Al-Gaadi B., Göktepe F., Halász M., Tamás P.:  Examination the drapability of textiles using ring-controlled equipment. ‘International Technical Textile Congress, Turkey, Istanbul’, 2010. 05. 16-18. 8 pp. (Poster and CD).
5. Al-Gaadi B.: 3D-s szkennelés alkalmazása a redőződés mérésére. Magyar Textiltechnika (in Hungarian), 2, 56-59 (2010).
6. Al-Gaadi B., Halász M., Tamás P.: Textiles dynamically influenced drapability. Materials Science Forum, 659, 361-366 (2010).
7. Al-Gaadi B., Halász M., Tamás P.: Textíliák dinamikusan befolyásolt redőződése. ’Országos Anyagtudományi Konferencia, Balatonkenese’,(in Hungarian) 2009. 10. 11-13. (Poster).
8. Tamás P., Göktepe F., Halász M. and Al-Gaadi B.: New method for dynamic drape measurement of fabrics. ’AUTEX 2009 Conference, Turkey, Izmir’, 2009. 05. 25-28. 579-587 (Oral presentation and CD).

 

Links

 

Department of Polymer Engineering

BUTE Géza Pattantyús-Ábrahám Doctoral School

eXPRESS Polymer Letters journal

Woven fabric

Composite

Videoextesomete

References

 

1. Lomov S. V., Boisse P., Deluycker E., Morestin F., Vanclooster K., Vandepitte D., Verpoest I., Willems A.: Full-field strain measurements in textile deformability studies. Composites Part A: Applied Science and Manufacturing, 39, 1232-1244 (2008).
2. Potluri P., Parlak I., Ramgulam R., Sagar T. V.: Analysis of tow deformations in textile preforms subjected to forming forces. Composites Science and Technology, 66, 297-305 (2006).
3. Galliot C., Luchsinger R. H.: The shear ramp: A new test method for the investigation of coated fabric shear behaviour - Part I: Theory. Composites Part A: Applied Science and Manufacturing, 41, 1743-1749 (2010).
4. Mohammed U., Lekakou C., Bader M. G.: Experimental studies and analysis of the draping of woven fabrics. Composites Part A: Applied Science and Manufacturing, 31, 1409-1420 (2000).
5. Galliot C., Luchsinger R. H.: The shear ramp: A new test method for the investigation of coated fabric shear behaviour - Part I: Theory. Composites Part A: Applied Science and Manufacturing, 41, 1743-1749 (2010).
6. Cusick G. E.: The dependence of fabric drape on bending and shear stiffness. Journal of the Textile Institute, 56, 596-606 (1965).
7. Jevšnik S., Geršak J.: Modeling the fused panel for a numerical simulation of drape. Fibers and Textiles in Eastern Europe, 12, 47-52 (2004).
8. Tamás P., Geršak J., Halász M.: Sylvie 3D drape tester - New system for measuring fabric drape. Tekstil, 10, 497-502 (2006).
9. Prodromou A. G., Chen J.: On the relationship between shear angle and wrinkling of textile composite preforms. Composites Part A: Applied Science and Manufacturing, 28, 491-503 (1997).