![]()
Theory Group
![]()
|
|
Theory Group |
|
The numerical renormalization group method (NRG) was first introduced by Wilson in the late 70's to solve the Kondo problem. Later NRG became one of the most popular tools to solve quantum impurity problems: It can be used to compute dynamical correlation functions as well as thermodynamic quantities, and very recently it has also been used to evaluate time-dependent correlation functions.
To perform efficient NRG calculations, one needs to exploit the symmetries as much as possible. Furthermore, for more delicate problems it is also essential to use a density matrix approach combined with recent spectral sum-conserving methods.
The DM-NRG code developed in the theory group is available for the public (www.phy.bme.hu/~dmnrg) and distributed under the GNU LGPL. This license makes the code free to use, share, and improve, and allows you to pass on the resulting code. The license gives freedom, but also sets firm restrictions on the use with non-free programs. The code is one of the most efficient NRG codes: It combines the spectral sum-conserving methods of Weichselbaum and von Delft and of Peters, Pruschke and Anders (both relying upon the complete basis set construction of Anders and Schiller) with the use of non-Abelian symmetries in a flexible manner. Essentially any non-Abelian symmetry can be taught to the code, and any number of such symmetries can be used throughout the computation for any density of states, and to compute any local operators' correlation function's real and imaginary parts or any thermodynamical expectation value. The code works both at zero and finite temperatures.
|
|
|
Frequency-dependent spin current noise and spin conductance through a quantum dot in the local moment regime computed with the Budapest DMNRG code. Spin current correlations are shown to behave markedly differently from charge correlations: Equilibrium spin cross-correlations are suppressed at frequencies below the Kondo scale, and are characterized by a universal function that we determine numerically for T = 0 temperature. For asymmetrical quantum dots dynamical spin accumulation resonance is found at the Kondo energy, TK. At higher temperatures surprising low-frequency anomalies related to overall spin conservation appear. For the details, see [1]. |
The NRG method turned out to be very successful approach to solve quantum impurity models numerically. Recently it has been proven that the approximate eigenstates of the Hamiltonian provided by NRG are of the form of matrix product states. This observation established a link between NRG and Density Matrix Renormalization Group (DMRG) since the latter method was known previously as a variational method optimizing matrix product states.
The combination of those two methods allows one to solve quantum impurity models much more efficiently. As a result, it becomes feasible to study complex quantum impurity problems that would be very challenging for standard NRG.
Recently, we have started to develop an efficient VMPS code to widen the set of problems that we can treat computationally. We have benefited a lot from the intensive collaboration with A. Weichselbaum and J. von Delft, two pioneers of VMPS method.
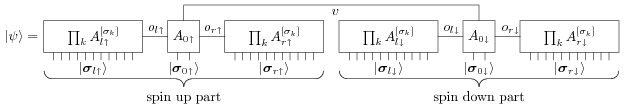 |
|
MPS representation for a quantum dot coupled to two spinful leads. Taken from A. Holzner et. al., Phys. Rev. B 81, 125126 (2010) |
The functional renormalization group method provides an efficient way to handle the interplay and/or competition between different energy scale correlations complementing the abilities of DMNRG and VMPS approaches. Practical applications of the method rely on truncations of the hyerarchy of the flow equations resulting in an effective action as a function of a continuously decreasing energy cutoff. The unique advantage of fRG is its semi-analytical nature which makes it easy to extend to more complex setups of quantum impurities.
Very recently we have started to use fRG aiming to treat more complex quantum dot setups with a special focus on their non-equilibrium properties.
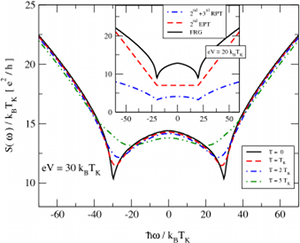 |
|
Temperature-dependence of the symmetrical noise, as computed by FRG. Inset: Comparison with third order renormalized perturbation theory (RPT). For the details, see [2]. |
[1] C. P. Moca, I. Weymann, G. Zaránd, Theory of frequency-dependent spin current noise through correlated quantum dots, Phys. Rev. B 81, 241305(R) (2010)
[2] C.P. Moca, P. Simon, C.H. Chung, G. Zaránd, Non-equilibrium frequency-dependent noise through a quantum dot: A real time functional renormalization group approach, arXiv:1008.0150 (2010).
-
Group of J. von Delft at LMU Munich, Germany
-
C.P. Moca at University of Oradea, Romania